Romps and Charn, Sticky thermals: Evidence for a dominant balance between buoyancy and drag in cloud updrafts, JAS, 2015
Paper
Description
Quickly rising clouds are associated with many important phenomena, including hail, turbulence, and lightning. But, what sets the speeds of these rising clouds? In other words, what is the balance of forces giving rise to the observed cloud updraft speeds? Here, we answer this question by studying thousands of cloud thermals in a high-resolution large-eddy simulation (LES) of deep convection.
Schematically, the acceleration of a cloud thermal can be written as dW/dt = B - D, where B is the buoyancy and D is the drag. (Note to fluid dynamicists: the virtual-mass correction is ignored in this schematic equation for simplicity.) The slippery-thermal hypothesis, advocated by Sherwood et al. (2013), states that the dominant balance in this equation is dW/dt = B. In other words, acceleration equals buoyancy. An alternative hypothesis, which we dub the sticky-thermal hypothesis, is that the dominant balance is B = D. In other words, drag balances buoyancy.
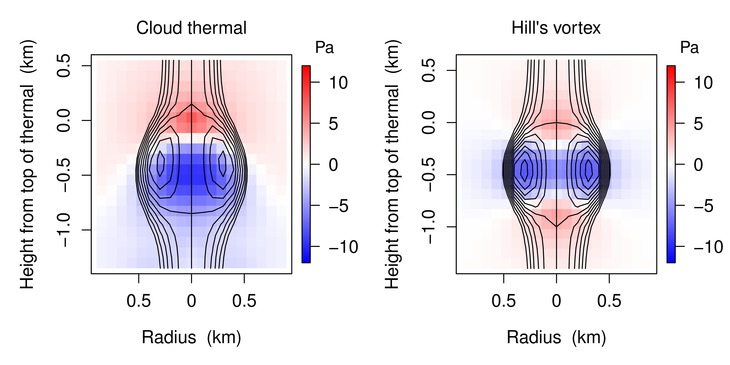
To identify which of these hypotheses is correct, we track thousands of cloud thermals in an LES of deep convection, average their properties around a vertical axis through their top, and identify the thermal's volume using its stream function. Averaging the bouyancy and the drag over the cloud thermal, we find that buoyancy and drag are in very close balance. In other words, cloud thermals are sticky.
Hill's vortex (shown on the right) is a classic steady-state solution to inviscid fluid dynamics. It is slippery thanks to the fore-aft symmetry in its pressure perturbations. Real cloud thermals do not have this symmetry. In fact, the symmetry is necessarily broken by their positive buoyancy, leading to asymmetric pressure perturbations and a net drag force.